MEAN RADIANT TEMPERATURE CALCULATOR
This tool calculates mean radiant temperature (MRT) and the percent people dissatisfied (PPD) from radiant asymmetry (RA) given the temperature of surfaces in a room, room dimensions, location of person and whether the person is sitting or standing.
These are important parameters to estimate the thermal comfort in a room, but are often challenging to calculate due to the complexities of calculating view factors between a person and the room surfaces.
This tool aims to simplify this calculation for most practical cases. Instructions on how to use the tool, some theoretical background, and an explanation of the underlying calculation methods can be found on this page.
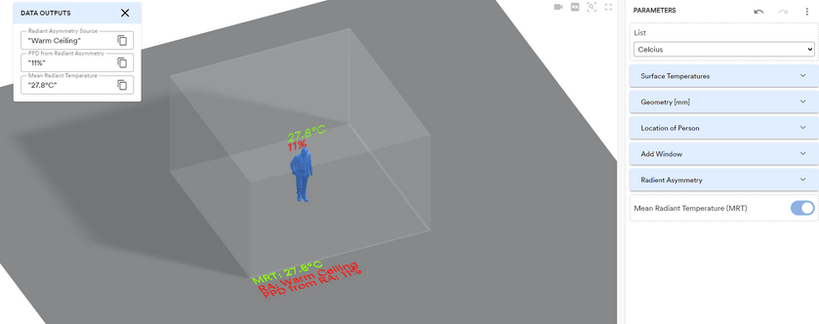
Fig. 1 Screenshot from calculation tool.
The calculator relies on user input for room geometry as well as surface temperatures. The input geometry and calculated results will be rendered in 3-dimensional space. The user can also add a window or enable calculation of percent dissatisfied from radiant asymmetry by toggling options under "Parameters".
The main unknown input is often surface temperatures. The table below can give some guiding input surface temperatures as a starting point for a critical condition for a typical building in Kuala Lumpur, Malaysia. Note that the temperature depends heavily on the specific case and should be adapted to the specific study.
Reference surface temperatures in Kuala Lumpur during day peak @ 32 degC outdoor air.
AC room is cooled to 24 degC.
Construction | Surface Temperature (non-AC) [degC] | Surface Temperature (AC) [degC] |
|---|---|---|
Insulated External Wall | 30 | 25 |
Non-insulated External Wall (Shaded) | 32 | 29 |
Internal Wall | 31 | 24 |
Floor/Ceiling | 31 | 24 |
Non-insulated External Ceiling (40% Reflective) | 41 | 40 |
Insulated External Ceiling | 31 | 26 |
Non-Insulated External Ceiling (80% Reflective) | 32 | 30 |
SGU Window (Shaded) | 36 | 31 |
DGU Window (Shaded) | 33 | 26 |
MEAN RADIANT TEMPERATURE (MRT)
Mean Radiant Temperature is the average temperature of all surfaces around a person adjusted for view factor.
For relatively low temperature differences and uniform surface emissivity this can be simplified to:
MRT = T1F1 + T2F2 + ... +TnFn
Where:
T: Surface temperature
F: View Factor between person and surface
In a building, radiating surfaces are typically ceilings, floors and walls, but could also be hot/cold windows or any internal item such as screens. The parameter describes the radiative heat exchange between a person and a room, unlike air temperature, which affects the convective heat exchange between a person and the air around.
Human skin experiences radiative and convective heat exchange differently, MRT is therefore a central parameter to predict the thermal comfort of a person in a room.
The thermal comfort of a room is typically quantified using either predicted mean vote (PMV) or percent people dissatisfied (PPD) as implemented using ASHRAE-55 method or EN-16798 method, which both takes MRT into account as either part of the operative temperature or as a direct input. A free thermal comfort calculator is available at CBE Thermal Comfort Tool.
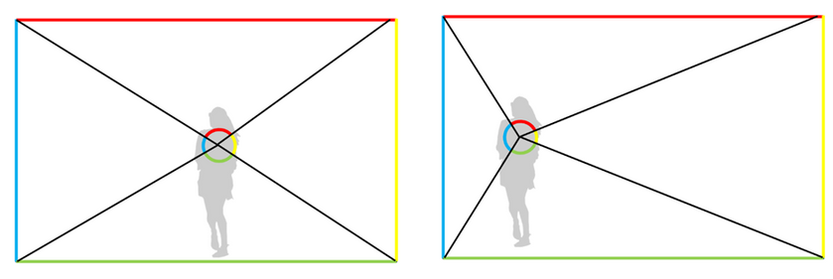
Fig. 2 View factor calculation concept simplified to 2d. The calculation tool extends this to 3d (Left) Person standing in the centre of the room will have uniform view factors to room surfaces. (Right) View factor adjusts if a person stands closer to a wall or the room geometry changes.
To predict MRT correctly, the view factor and radiant heat exchange to the room must be
modelled independently for each small patch of skin. This can be done using numerical methods such as raytracing, modelling the radiation ray by ray, which is very computationally heavy and difficult for complex geometries. The problem is therefore typically simplified for practical situations.
The method implemented in this tool is limited to a person within a rectangular box.
The person is represented by a sphere at chest height. The room is segmented by drawing lines from each corner. to the center of the sphere. The sphere is split into sub-surfaces by the lines. The view factor to each part of the room is the ratio of the area of the subsurface of the sphere to the area of the whole sphere. (Fig. 2)
Special care should be given if the room has any low-e surfaces. One of the base assumptions is that all materials have an emissivity of 0.9. This is no longer true with a low-e surface such as a ceiling treatment or low-e glass. In such cases the effective temperature of low-e surface should be estimated based on the specific situation which will differ from the actual temperature.
RADIANT ASYMMETRY (RA)
If the differences in temperatures between surfaces in the room becomes large, people in the room will experience thermal discomfort from the contrast in sensations on various parts of the body. This is defined as the difference in MRT on opposing sides of the body.
The connection between radiant asymmetry and thermal comfort is described in Fig. 3 as percent people dissatisfied (PPD) as a function of radiant asymmetry.
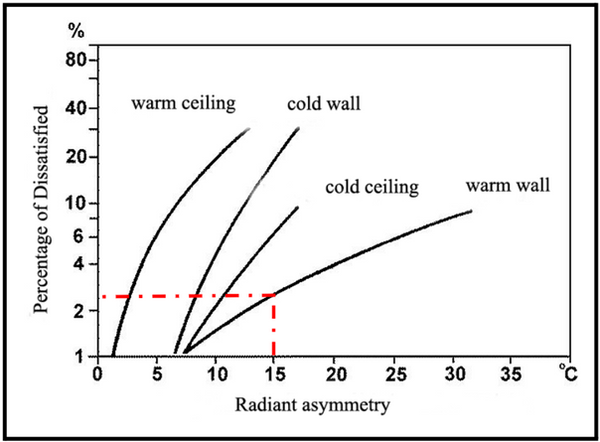
Fig. 3 Relationship between thermal comfort and radiant asymmetry - Here for a warm wall
For hot climates this effect is clearest for warm ceilings (or directly under the sun) which frequently happens for poorly insulated roofs.
The radiant asymmetry is implemented in the tool based on the calculated MRT.
The average MRT from surfaces above the centreline of the reference sphere is subtracted from the average MRT from surfaces below the centreline of the reference sphere (Fig. 4) to get the radiant asymmetry. The resulting temperature difference is then used to get estimate the predicted mean vote using the graph in fig. 3. Whether a surface is considered warm or cold is decided whether it is hotter or colder than the ambient room temperature.
The procedure is then repeated for each wall and the worst PPD and critical condition is returned to the user.

Fig. 4 Concept for calculation of radiant asymmetry. The process is repeated for all walls and the worst situation is identified.
